Things to Learn:

  • Understand that two quantities are proportional to each other when there exists a constant (number) such that each measure in the first quantity multiplied by this constant gives the corresponding measure in the second quantity.
  • Identify the measures in the first quantity with `x` and the measures in the second quantity with `y`, and recognize that the second quantity is proportional to the first quantity if `y = kx` for some positive number `k`. Apply this same relationship when using variable choices other than `x` and `y`.

Yogurt by the Ounce


This Desmos Calculator table and graph will show the relationship of the weight and cost of the yogurt. (Click the icon for the full calculator).

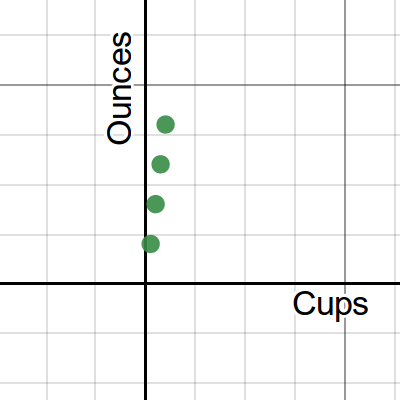
1.2 Example 2: A Cooking Cheat Sheet:

Mr. Clarkson will show you how to set up a Desmos Calculator table. Use this link to open a calculator. Add the values for 4 cups, 6 cups, and 8 cups.

Lesson Summary

Measures of one type of quantity are proportional to measures of a second type of quantity if there is a number k so that for every measure x of a quantity of the first type, the corresponding measure y of a quantity of the second type is given by kx; that is, y = kx. The number k is called the constant of proportionality.

A proportional relationship is a correspondence between two types of quantities such that the measures of quantities of the first type are proportional to the measures of quantities of the second type.

Note that proportional relationships and ratio relationships describe the same set of ordered pairs but in two different ways. Ratio relationships are used in the context of working with equivalent ratios, while proportional relationships are used in the context of rates.

Exercises and Practice Click the link to do the homework.